2. 青岛海洋科学与技术试点国家实验室海洋渔业科学与食物;
4. 中国海洋大学水产学院 青岛 266003
2. Laboratory for Marine Fisheries Science and Food Production Processes, Pilot National Laboratory for Marine Science and Technology (Qingdao), Qingdao 266071;
3. Key Laboratory of Sustainable Development of Marine Fisheries, Ministry of Agriculture and Rural Affairs, Shandong Provincial Key Laboratory of Fishery Resources and Eco-Environment, Yellow Sea Fisheries Research Institute, Chinese Academy of Fishery Sciences, Qingdao 266071;
4. College of Fisheries, Ocean University of China, Qingdao 266003
为了探究变量在不同空间尺度上的分布格局和变化规律,通常要求采样信息具有空间上的连续性,但是受成本和技术等限制,对于高山、地层和海洋等难以到达或范围较大的研究区域,只能获取具有一定分辨率的分散样本点数据(Li et al, 2014),空间插值法可以利用有限的数据,对整个研究区域的变量进行预测,减少采样调查的费用和难度,因此,被广泛应用于水文、气象、土壤、矿产、农业和生态等方面(孙铭帅等, 2017; 岳文泽等, 2005)。空间插值法可以分为非地统计法、地统计法和二者结合的方法(Li et al, 2014)。受自身算法、数据本身属性以及样本点数量等多种因素的影响,不同情况下空间插值法的精度存在一定差异(朱会义等, 2004),例如,孙慧等(2017)研究发现,Cubist方法预测的土壤Cd含量结果精度较高;Chen等(2016)发现,不同采样时间对渔业资源密度空间插值的精度存在差异;Luo等(2011)对多种方法估算的月降水量的空间分布进行比较发现,协同克里格法(Ordinary cokriging, COK)的精度要高于泰森多边形法、反距离加权法(Inverse distance weighted, IDW)和普通克里格法(Ordinary kriging, OK)。因此,在实际应用时,有必要先对不同插值方法进行比较分析,选择最优的方法。
物种多样性在不同尺度上的空间分布特征是生态学研究中的重要问题(李圣法, 2005; 马斌等, 2008)。游泳动物作为水生生物群落的重要组成部分,其多样性空间变化可以在一定程度上反映物种多样性的变动趋势。目前,只在陆地生物多样性空间分布中发现不同空间插值法的比较研究(Hernandez-Stefanoni et al, 2006),虽然空间插值法在海洋生物多样性空间分布中已有应用(Stelzenmüller et al, 2009;Tittensor et al, 2010; 张敏等, 2011; 吕振波等, 2012),但是尚未见不同方法优劣的判别研究。因此,本研究比较分析了5种常用的空间插值法[包括IDW、径向基函数法(Radial basis function, RBF)、局部多项式法(Local polynomial interpolation, LPI)、OK和COK]对4种多样性指数[即物种种类数(S)、Shannon-Weaver多样性指数(H’)、Pielou均匀度指数(J)和Margalef丰富度指数(d)]的插值结果,选出最适合海洋游泳动物多样性插值的方法,以期为海洋生态学研究提供参考。
1 数据与方法 1.1 数据来源数据来自2017年5月黄海和东海北部渔业资源底拖网调查,调查站位见图 1。调查船为中国水产科学研究院黄海水产研究所“北斗”号,平均拖速为3.0 kn,拖网时间为0.5~1 h,调查网具网目尺寸为836目×20 cm,囊网网目为2.4 cm,网具总长度为83.2 m。渔获物鉴定到种,并记录每一个种类的尾数和重量。数据分析前,将原始数据标准化为1 h的渔获尾数和渔获重量。

|
图 1 黄海和东海北部调查站位图 Fig.1 Survey stations in the Yellow Sea and north East China Sea |
采用物种种类数(S)、Shannon-Weaver多样性指数(H′)、Pielou均匀度指数(J)和Margalef丰富度指数(d)对游泳动物多样性进行分析,公式如下:
| $ {H^{'}} = - \sum\limits_{i = 1}^S {{P_i}\ln {P_i}} $ |
| $ J = {H^{'}}/({\rm ln}S) $ |
| $ d = (S - 1)/{\rm ln}N $ |
式中,S为每个站位游泳动物种类数,Pi为第i种游泳动物重量占总重量的比例,N为所有游泳动物的总重。
1.3 空间插值法 1.3.1 反距离加权插值IDW基于相近相似原理(Chaplot et al, 2006),假设每一个样本点的值都会对邻近区域产生影响,距离未知点越近的样本点对其影响程度越大。公式如下(Shepard, 1968):
| $ Z({x_0}) = \sum\limits_{i =1}^n {d_i^{ - w}Z({x_i})/} \sum\limits_{i = 1}^n {d_i^{ - w}} $ |
式中,
RBF是利用径向基函数逼近样本点的值,产生一个贯穿所有样本点且总曲率最小的曲面(Powell, 1981; Xie et al, 2011)。公式如下:
| $ Z({x_0}) = \sum\limits_{i = 1}^n {\alpha ({x_i})\phi (\left\| {x - {x_i}} \right\|)} $ |
式中,x为某一样本点;
| $ {\alpha ^T}({x_i}) = \{ {\alpha _1}, {\alpha _2}, ..., {\alpha _n}\} $ |
式中,
LPI是利用观测数据拟合出的多项式方程对未知点进行预测,其中,样本点的位置作为自变量,观测值作为因变量。一阶多项式生成的预测图为平面,二阶多项式生成的预测图有1个曲面,依次类推。
1.3.4 普通克里格插值OK是利用样本的半变异函数模型的结构特点,对未知点进行线性无偏最优估计的过程(Krige, 1953),通常在期望未知的情况下使用,并且要求数据符合正态分布。公式如下:
| $ Z({x_0}) = \sum\limits_{i= 1}^n {\lambda ({x_i})Z({x_i})} $ |
式中,
| $ r(h) = \frac{1}{{2N(h)}}\sum\limits_{i= 1}^{N(h)} {{{(Z({x_i}) - Z({x_i} + h))}^2}} $ |
式中,r(h)为距离等于h时变量的半方差函数;N(h)为距离等于h时点对的个数;
以统计学上的无偏性和最优性为条件,结合拉格朗日原理,可推导出权重值和半方差函数之间关系的公式(王政权, 1999)。
1.3.5 协同克里格插值COK是利用主变量之间的相关性及其与辅助变量的交互相关性,优化插值的精度(姜勇等, 2006),常用于主变量数据难以获取或直接用主变量插值的结果不准确的情况(李润林等, 2013)。公式如下(Myers, 1982):
| $ Z({x_0}) = \sum\limits_{i= 1}^n {\lambda ({x_i})Z({x_i})} + \sum\limits_{j = 1}^n {\lambda ({x_j})Z({x_j})} $ |
式中,
进行OK和COK之前,数据必须符合正态分布,因此,对上文计算的4种物种多样性指数数据进行Shapiro-Wilk检验(Chen et al, 2016)。若不满足正态分布,则进行数据转换。因为数据结构存在差异,本研究采用BOX-COX和平方2种转换方式(于洋等, 2015; 章清等, 2016)。
1.5 效果评价采用jackknife检验比较不同空间插值法的预测效果。该检验的主要步骤为:首先,对于已有的n个样本,依次去除其中1个样本点,利用剩余的n-1个样本作为训练集对去除点进行估算,重复n次,得到所有样本点的预测值;其次,计算预测值与样本实际观测值的决定系数(Coefficient of determination, R2)、平均绝对误差(Mean absolute error, MAE)和均方根误差(Root mean square error, RMSE)。R2反映样本值和预测值之间的相关程度,R2越接近1,表示该空间插值法的预测值与样本值的变化趋势越相似,反之则偏差越大;MAE可以反映预测值可能的误差范围;MAE和RMSE都可以反映插值结果的精度,且都是越小越好(林忠辉等, 2002; 董志南等, 2015; 于洋等, 2015)。公式如下:
| $ R = \frac{{\sum\limits_{i= 1}^n {({Z^*}({x_i}) - \overline {{Z^*}({x_i})})(Z({x_i}) - \overline {Z({x_i})} } }}{{\sqrt {\sum\limits_{i= 1}^n {{{({Z^*}({x_i}) - \overline {{Z^*}({x_i})})}^2}} } \sqrt {\sum\limits_{i= 1}^n {{{(Z({x_i}) - \overline {Z({x_i})})}^2}} } }} $ |
| $ {\rm{MAE = }}\frac{1}{n}\sum\limits_{i= 1}^n {\left| {{Z^*}({x_i}) - Z({x_i})} \right|} $ |
| $ {\rm{RMSE = }}\sqrt {\frac{1}{n}\sum\limits_{i= 1}^n {{{({Z^*}({x_i}) - Z({x_i}))}^2}} } $ |
式中,
物种多样性指数计算及其描述性统计、MAE及R2的计算、数据的正态分布检验和转换采用R3.5.2完成;半方差函数的拟合采用GS+7.0软件完成,因GS+7.0软件无法计算具有多个辅助变量条件下的半方差函数,因此,COK的半变异函数中的C0、C和C0/C 3个参数是未知的,其变程的计算是由ArcGIS10.2完成;4种多样性指数之间的相关性判断采用SPSS17.0完成;坐标转换、空间插值和交叉验证采用ArcGIS10.2完成。
2 结果 2.1 数据的统计描述4种多样性指数的描述性统计见表 1,Shpiro-Wilk正态检验发现,S、J和d属于偏态分布(P < 0.05)。根据数据特点,对S和d进行BOX-COX转换,对J进行平方转换,转换前对数据进行加1处理。转换后,数据均通过Shpiro-Wilk正态检验(P > 0.1),符合正态分布。此外,H′符合正态分布(P > 0.05),不做转换。
|
|
表 1 物种多样性指数描述性统计和Shapiro-Wilk检验的P值 Tab.1 Descriptive statistics of five species diversity indices and P-values of the Shapiro-Wilk test |
表 2列举了物种多样性指数之间的相关性,其中,S、H′和d之间具有极显著的正相关关系。J与H′呈极显著正相关关系,与d呈显著正相关,而与S相关性不显著。将具有极显著相关的物种多样性指数作为辅助变量,当存在多个具有显著相关的辅助变量时,根据ArcGIS10.2交叉验证的结果初步进行筛选。S和J最适合的辅助变量均为H′和d;H′最适合的辅助变量为S和J;d最适合的辅助变量为S、H′和J。
|
|
表 2 物种多样性指数之间的相关性 Tab.2 Correlations among the species diversity indices |
根据GS+7.0软件的拟合结果(表 3),不同物种多样性指数的半方差函数适合不同的模型。S的空间分布为中等空间相关性(C0/C=0.43),其他3个指数均为强空间相关性(C0/C < 0.25),符合空间插值的前提。不同物种多样性指数对应的变程差别较大:S和d的变程较大,说明其在大范围内的空间自相关性较强,H′和J的变程都较小,说明在小尺度范围内二者的空间自相关性较强。
|
|
表 3 物种多样性指数的半方差函数及相关参数 Tab.3 Semivariance models and the corresponding parameters of species diversity indices |
对于S,5种空间插值法按预测值与样本值的R2大小排序为LPI < IDW < OK < RBF < COK;按MAE和RMSE大小排序的结果相同,为COK < RBF < OK < IDW < LPI。对于H′,5种空间插值法按预测值与样本值的R2大小排序为IDW < RBF < OK < LPI < COK;按MAE大小排序为COK < RBF < OK < IDW < LPI;按RMSE大小排序为COK < LPI < RBF < IDW < OK。对于J,5种空间插值法的预测值与样本值的R2大小排序为RBF < IDW < OK < LPI < COK;按MAE大小排序为COK < RBF < OK < LPI < IDW;按RMSE大小排序为COK < OK < RBF < IDW < LPI。对于d,5种空间插值法按预测值与样本值的R2大小排序为IDW < LPI < RBF < OK < COK;MAE大小排序和RMSE相同,为COK < OK < RBF < IDW < LPI(表 4)。由此可以看出,对于4种多样性指数,COK的结果最精确,其他4种方法精度差别较小且很低。
|
|
表 4 不同插值方法的交叉验证结果 Tab.4 Cross-validation diagnostic of different interpolation methods |
4种多样性指数在样本点的大小分布与预测的空间分布基本一致,在非采样区域的曲线平滑度都低于采样区域(图 2)。S和d整体的空间分布格局相似,都呈现出明显的东南部高,北部次之,中部最低的格局。H′和J也是中部最低,但是在南部和北部海域差异不明显。4种多样性指数在32°N以南海域高值区的范围大致相同。
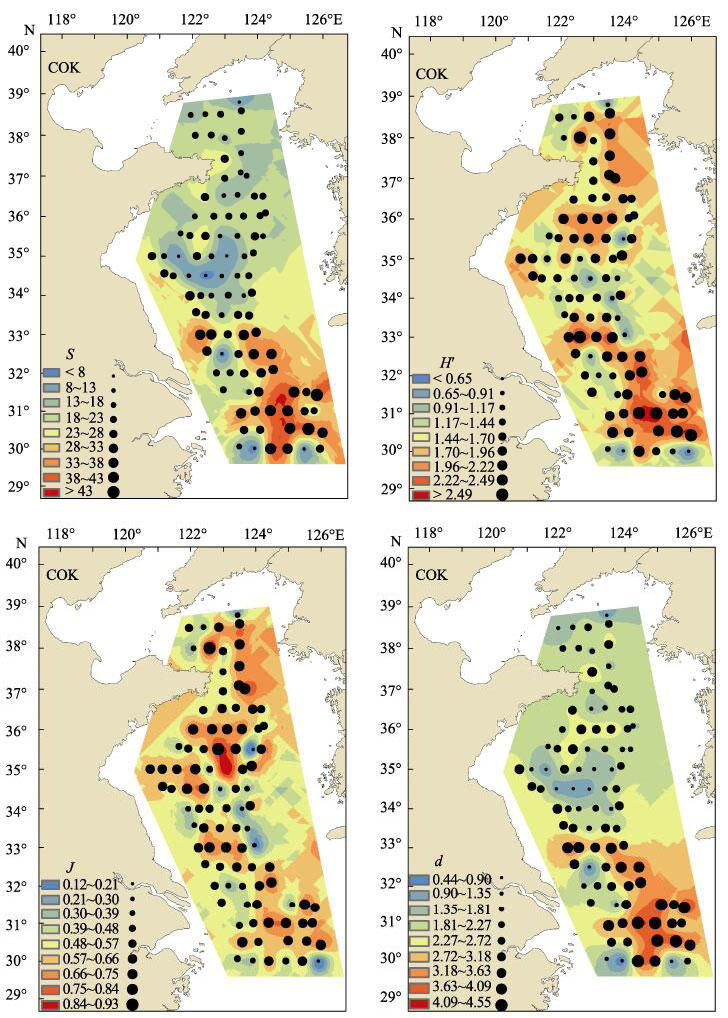
|
图 2 协同克里格插值(COK)预测出的物种种类数(S)、Shannon-Weaver多样性指数(H′)、Pielou均匀度指数(J)和Margalef丰富度指数(d)的空间分布图 Fig.2 Spatial distribution of number of species (S)、Shannon-Weaver diversity index (H′)、Pielou′s evenness index (J) and Margalef′s richness index (d) by ordinary cokriging (COK) |
分析COK的预测图,在大尺度上,2017年春季黄海和东海北部游泳动物的S和d大致呈现明显的南高北低的格局,而H′和J的南北差异不大。与台湾海峡游泳动物多样性(宋普庆等, 2012)以及相邻海域鱼类群落多样性(程济生, 2000)相比,除了d具有相同的空间格局,后2种多样性指数空间分布格局均有较大差别。在小尺度上,34°~35.5°N,121°~124°E附近海域的S和d明显比周围海域低,而H′和J在此范围内没有明显的低值区。在30°~32°N范围内的海域中,4种多样性指数均有高值区,而且西部海域均低于东部海域。在刘勇等(2017)的研究中,30°~32°N海域底层渔业生物的S和d也存在高值区,而且S、H′和d三者也呈现东部海域高于西部海域的格局,但是J在124°~ 126°E之间海域存在大范围的低值区,与本研究结果有较大差别。
游泳动物多样性指数空间分布变化特征可能与环境、优势种、饵料丰度及分布、栖息地状况、人为活动和鱼类洄游行为等多种因素有关(Jin et al, 1996; 戴芳群等, 2020; 金显仕等, 2009; 程济生等, 2004; 朱鑫华等, 1994)。从水文环境方面看,因为多样性指数与环境因子的相关关系可以量化,而且数据容易获取,常受到人们关注。已有研究表明,春季黄海中南部的S和d与海底水温显著正相关(P < 0.05),与深度、海表温度和盐度关系不显著(P > 0.05)(单秀娟等, 2014),说明物种多样性的空间分布可能受海底水温影响程度较大;而H’和J与深度、海底和海表的水温和盐度的关系不密切(单秀娟等, 2014)。受海底地形、海流等因素影响,在春、夏和秋季,黄海中北部海域存在一个稳定且大范围的冷水团(李洪波等, 2006; 于非等, 2006; 王家栋等, 2011)。而在2017年春季,35°N,122°~124°E附近海域的海底温度最高为10℃,低于附近海域的温度(孔凡洲等, 2018)。这可能是导致4种多样性指数在大尺度上以及34°~35.5°N,122°~124°E附近海域呈现独特的分布特点和差异的原因。对于30°~32°N范围内的东海北部海域,多样性指数较低的原因可能是靠近长江口受淡水冲击导致附近海域环境条件不稳定,海水浊度较高,不利于生物生活(Whitfield, 1994; 刘淑德等, 2009, 徐勇等, 2014)。从繁殖特性方面看,春季是很多游泳动物繁殖的季节,繁殖群体会洄游到环境适宜的海域产卵。如位于32°~34°N、122.5°E以西海域的吕四渔场是银鲳(Pampus argenteus)、小黄鱼(Larimichthys ployactis)和黄鲫(Setipinna tenuifilis)等种类主要产卵场(陈新军, 2004; 刘勇等, 2017)。在30°~32.5°N,123°~126°E之间的海域存在多个重要渔场,例如,舟山渔场、江外渔场和舟外渔场等,是多种重要渔业种类的产卵场(刘勇等, 2007、2014; 林龙山等, 2008)。影响游泳动物空间分布的原因复杂多变,对于其他小尺度上的多样性指数空间特征形成原因,还需要进一步研究和分析。
3.2 空间插值法分析插值结果的精度取决于空间插值模型对变量空间变异性和相关性的解释度(朱会义等, 2004)。本研究中,4种多样性指数的COK jackknife检验结果都比较好,解释度高于其他方法,这与很多研究结果一致(卢月明等, 2017; 李润林等, 2013; 石朋等, 2005; 岳文泽等, 2005)。但是,在日降水量(宋丽琼等, 2008)和热带雨林植物多样性(Hernandez-Stefanoni et al, 2006)的空间插值法比较研究中,COK的精度要低于OK。根据王平等(2014)对不同辅助变量的COK插值结果比较,发现当主变量和辅助变量之间的相关性大于0.45时,COK精度才会明显高于OK。可见,辅助变量的选择是COK插值的关键步骤,合适的辅助变量可以优化COK。目前,最简单的方法是直接将与主变量相关性较高的因子作为辅助变量。如果主变量有多个显著相关的因子,可以根据ArcGIS软件交叉验证的结果进行初步筛选。但是,当与主变量显著相关的因子数量过多时,这种筛选方式会导致工作量增加,而且单一的辅助变量可能对模型优化程度有限(章清等, 2016)。因此,基于主成分分析的COK受到广泛关注。这种方法是利用主成分分析,将多个与主变量显著相关的因子赋予不同的权重,再合并成综合指标作为辅助变量(卢月明等, 2017),在鱼类资源丰度和土壤要素的空间分布研究中都取得了较好的结果(方学燕等, 2017; 章清等, 2016)。
3.3 展望本研究采用了多个辅助变量,主变量与辅助变量之间的相关性很高,因此,相对于OK,COK的精度有了极大提高。研究表明,S、H′、J和d之间存在较高的相关性,这种现象在不同季节和年份同样存在(单秀娟等, 2014),因此,COK不仅可以用于分析和预测春季黄海和东海北部游泳动物多样性空间分布,还可能适用于不同时间维度。在今后的研究中,可以结合与游泳动物多样性有显著关系的环境因子,并基于主成分分析法减少辅助变量的数量,尝试进一步提高COK的精度。
Chaplot V, Darboux F, Bourennane H, et al. Accuracy of interpolation techniques for the derivation of digital elevation models in relation to landform types and data density. Geomorphology, 2006, 77(1-2): 126-141 DOI:10.1016/j.geomorph.2005.12.010 |
Chen XJ. Fishery resources and fishing ground. Beijing: China Ocean Press, 2004: 266-267
|
陈新军. 渔业资源与渔场学. 北京: 海洋出版社, 2004: 266-267
|
Chen Y, Shan X, Jin X, et al. A comparative study of spatial interpolation methods for determining fishery resources density in the Yellow Sea. Acta Oceanologica Sinica, 2016, 35(12): 65-72 DOI:10.1007/s13131-016-0966-y |
Cheng JS, Yu LF. The change of structure and diversity of demersal fish communities in the Yellow Sea and East China Sea in winter. Journal of Fisheries of China, 2004, 28(1): 29-34 |
程济生, 俞连福. 黄、东海冬季底层鱼类群落结构及多样性变化. 水产学报, 2004, 28(1): 29-34 |
Cheng JS. The structure and diversity of demersal fish communities in winter in the East China Sea and the Yellow Sea. Marine Fisheries Research, 2000, 21(3): 1-8 |
程济生. 东、黄海冬季底层鱼类群落结构及其多样性. 海洋水产研究, 2000, 21(3): 1-8 |
Dong ZN, Zheng SN, Zhao HB, et al. Comparative analysis of methods of wind field simulation based on spatial interpolation. Journal of Geo-Information Science, 2015, 17(1): 37-44 |
董志南, 郑拴宁, 赵会兵, 等. 基于空间插值的风场模拟方法比较分析. 地球信息科学学报, 2015, 17(1): 37-44 |
Dai FQ, Zhu L, Chen YL. Variations of fishery resource structure in the Yellow Sea and East China Sea. Progress in Fishery Sciences, 2020, 41(1): 1-10 |
戴芳群, 朱玲, 陈云龙. 黄、东海渔业资源群落结构变化研究. 渔业科学进展, 2020, 41(1): 1-10 |
Fang XY, Chen XJ, Feng YJ, et al. Study of spatial distribution for Dosidicus gigas abundance off Peru based on a comprehensive environmental factor. Haiyang Xuebao, 2017, 39(2): 62-71 |
方学燕, 陈新军, 冯永玖, 等. 基于综合环境因子的协同克里金法分析茎柔鱼资源丰度空间分布. 海洋学报, 2017, 39(2): 62-71 |
Feng YJ, Fang XY, Chen XJ, et al. Spatial interpolation and uncertainty of neon flying squid (Ommastrephes bartramii) resources in the Northwest Pacific Ocean. Recourses Science, 2015, 37(11): 2299-2308 |
冯永玖, 方学燕, 陈新军, 等. 基于GIS的西北太平洋柔鱼资源空间插值及不确定性分析. 资源科学, 2015, 37(11): 2299-2308 |
Guo XD, Fu BJ, Chen LD, et al. The spatio-temporal variability of soil nutrients in Zunhua Plain of Hebei Province: Semivariogram and Kriging analysis. Acta Geographica Sinica, 2000, 55(5): 555-566 |
郭旭东, 傅伯杰, 陈利顶, 等. 河北省遵化平原土壤养分的时空变异特征—变异函数与Kriging插值分析. 地理学报, 2000, 55(5): 555-566 |
Hernandez-Stefanoni JL, Ponce-Hernandez R. Mapping the spatial variability of plant diversity in a tropical forest: Comparison of spatial interpolation methods. Environmental Monitoring and Assessment, 2006, 117(1-3): 307-334 DOI:10.1007/s10661-006-0885-z |
Jiang Y, Li Q, Zhang XK, et al. Kriging prediction of soil zinc in contaminated field by using an auxiliary variable. Chinese Journal of Applied Ecology, 2006, 17(1): 97-101 |
姜勇, 李琪, 张晓珂, 等. 利用辅助变量对污染土壤锌分布的克里格估值. 应用生态学报, 2006, 17(1): 97-101 |
Jin XS, Tang QS. Changes in fish species diversity and dominant species composition in the Yellow Sea. Fisheries Research, 1996, 26(3-4): 337-352 DOI:10.1016/0165-7836(95)00422-X |
Jin XS, Shan XJ, Guo XW, et al. Community structure of fishery biology in the Yangtze River estuary and its adjacent waters. Acta Ecologica Sinica, 2009, 29(9): 4761-4772 |
金显仕, 单秀娟, 郭学武, 等. 长江口及其邻近海域渔业生物的群落结构特征. 生态学报, 2009, 29(9): 4761-4772 |
Jing YP, Zhang SW, Li Y. Landscape pattern and SHDI spatial structure characteristics of rural-urban fringe: A case study in Jingyue development zone in Changchun City. Recourses Science, 2007, 29(5): 43-49 |
荆玉平, 张树文, 李颖. 城乡交错带景观格局及多样性空间结构特征—以长春净月开发区为例. 资源科学, 2007, 29(5): 43-49 |
Kong FZ, Jang P, Wei CJ, et al. Co-occurrence of green tide, golden tide and red tides along the 35°N transect in the Yellow Sea during spring and summer in 2017. Oceanologia et Limnologia Sinica, 2018, 49(5): 1021-1030 |
孔凡洲, 姜鹏, 魏传杰, 等. 2017年春、夏季黄海35°N共发的绿潮、金潮和赤潮. 海洋与湖沼, 2018, 49(5): 1021-1030 |
Krige DG. A statistical approach to some basic mine valuation problems on the Witwatersrand. OR, 1953, 4(1): 18 |
Li HB, Xiao T, Ding T, et al. The distribution of bacterioplankton in the Yellow Sea Cold Water Mass (YSCWM). Acta Ecologica Sinca, 2006, 26(4): 1012-1020 DOI:10.1016/S1872-2032(06)60020-6 |
李洪波, 肖天, 丁涛, 等. 浮游细菌在黄海冷水团中的分布. 生态学报, 2006, 26(4): 1012-1020 |
Li J, Heap AD. Spatial interpolation methods applied in the environmental sciences: A review. Environmental Modelling and Software, 2014, 53: 173-189 DOI:10.1016/j.envsoft.2013.12.008 |
Li RL, Yao YM, Tang PQ, et al. Optimization of spatial interpolation and sampling size for soil zinc content in cultivated land at county scale using Cokriging method. Chinese Journal of Soil Science, 2013, 44(4): 830-838 |
李润林, 姚艳敏, 唐鹏钦, 等. 县域耕地土壤锌含量的协同克里格插值及采样数量优化. 土壤通报, 2013, 44(4): 830-838 |
Li SF. The ecological study of fish community in the East China Sea continental shelf: The spatial pattern and diversity. Doctoral Dissertation of East China Normal University, 2005
|
李圣法.东海大陆架鱼类群落生态学研究—空间格局及其多样性.华东师范大学博士研究生学位论文, 2005
|
Lin LS, Cheng JH, Jiang YZ, et al. Spatial distribution and environmental characteristics of the spawning grounds of small yellow croaker in the southern Yellow Sea and the East China Sea. Acta Ecologica Sinica, 2008, 28(8): 3485-3494 |
林龙山, 程家骅, 姜亚洲, 等. 黄海南部和东海小黄鱼(Larimichthys polyactis)产卵场分布及其环境特征. 生态学报, 2008, 28(8): 3485-3494 |
Lin ZH, Mo XG, Li HX, et al. Comparison of three spatial interpolation methods for climate variables in China. Acta Geographica Sinica, 2002, 57(1): 47-56 |
林忠辉, 莫兴国, 李宏轩, 等. 中国陆地区域气象要素的空间插值. 地理学报, 2002, 57(1): 47-56 |
Liu AL, Wang PF, Ding YY. An introduction to geostatistics. Beijing: Science Press, 2012
|
刘爱利, 王培法, 丁园圆. 地统计学概论. 北京: 科学出版社, 2012
|
Liu SD, Xian WW. Temporal and spatial patterns of the ichthyoplankton community in the Yangtze Estuary and its adjacent waters. Biodiversity Science, 2009, 17(2): 151-159 |
刘淑德, 线薇微. 长江口及其邻近水域鱼类浮游生物群落的时空格局. 生物多样性, 2009, 17(2): 151-159 |
Liu Y, Cheng JH. Temporal and spatial distribution of bottom fishery biodiversity in the middle and north of the East China Sea and the south of the Yellow Sea. Journal of Fisheries of China, 2017, 41(8): 1223-1236 |
刘勇, 程家骅. 东海中、北部及黄海南部海域底层渔业生物多样性时空分布特征. 水产学报, 2017, 41(8): 1223-1236 |
Liu Y, Cheng JH. Study on the correlation between spatial distributions of the spawning groups and the power b in length-weight relation function of small yellow croaker (Larimichthys polyactis). Acta Oceanologica Sinica, 2014, 36(6): 124-130 |
刘勇, 程家骅. 小黄鱼Larimichthys polyactis体长-体重关系幂指数与产卵群体空间分布相关性研究. 海洋学报, 2014, 36(6): 124-130 |
Liu Y, Yan LP, Cheng JH. Distribution of small yellow croaker′s spawner stocks and its relationship with seawater temperature and salinity in the open sea of northern East China Sea and southern Yellow Sea in 2003. Journal of Fishery Sciences of China, 2007, 14(7): 89-96 |
刘勇, 严利平, 程家骅. 2003年东海北部和黄海南部外海小黄鱼产卵群体的分布特征及其与水温、盐度的关系. 中国水产科学, 2007, 14(7): 89-96 |
Lu YM, Wang L, Qiu AG, et al. A CoKriging interpolation method based on principal component analysis. Bulletin of Surveying and Mapping, 2017(11): 51-57, 63 |
卢月明, 王亮, 仇阿根, 等. 一种基于主成分分析的协同克里金插值方法. 测绘通报, 2017(11): 51-57, 63 |
Luo X, Xu Y, Shi Y. Comparison of interpolation methods for spatial precipitation under diverse orographic effects. 2011 19th International Conference on Geoinformatics. IEEE, 2011, 1–5
|
Lü ZB, Li F, Xu BQ, et al. Fish community diversity during spring and autumn in the Yellow Sea off the coast of Shandong. Biodiversity Science, 2012, 20(2): 207-214 |
吕振波, 李凡, 徐炳庆, 等. 黄海山东海域春、秋季鱼类群落多样性. 生物多样性, 2012, 20(2): 207-214 |
Ma B, Zhou ZY, Zhang LL, et al. The spatial distribution characteristics of plant diversity in Alex Left Banner. Acta Ecologica Sinca, 2008, 28(12): 6099-6106 |
马斌, 周志宇, 张莉丽, 等. 阿拉善左旗植物物种多样性空间分布特征. 生态学报, 2008, 28(12): 6099-6106 |
Myers DE. Matrix formulation of co-kriging. Journal of the International Association for Mathematical Geology, 1982, 14(3): 249-257 DOI:10.1007/BF01032887 |
Powell MJD. Approximation theory and methods. Cambridge: Cambridge university press, 1981
|
Shan XJ, Chen YL, Dai FQ, et al. Variations in fish community and diversity in the sections of the central and southern Yellow Sea. Acta Ecologica Sinica, 2014, 34(2): 377-389 |
单秀娟, 陈云龙, 戴芳群, 等. 黄海中南部不同断面鱼类群落结构及其多样性. 生态学报, 2014, 34(2): 377-389 |
Shepard D. A two-dimensional interpolation function for irregularly-spaced data. Proceedings of the 1968 23rd ACM national conference. ACM New York, 1968, 517–524
|
Shi P, Rui XF. Comparison and improvement of spatial rainfall interpolation methods. Journal of Hohai University (Natural Sciences), 2005, 33(4): 361-365 |
石朋, 芮孝芳. 降雨空间插值方法的比较与改进. 河海大学学报(自然科学版), 2005, 33(4): 361-365 |
Song LQ, Tian Y, Wu L, et al. On comparison of spatial interpolation methods of daily rainfall data: A case study of Shenzhen. Geo-Information Science, 2008, 10(5): 566-572 |
宋丽琼, 田原, 邬伦, 等. 日降水量的空间插值方法与应用对比分析—以深圳市为例. 地球信息科学, 2008, 10(5): 566-572 |
Song PQ, Zhang J, Lin LS, et al. Nekton species composition and biodiversity in Taiwan Strait. Biodiversity Science, 2012, 20(1): 32-40 |
宋普庆, 张静, 林龙山, 等. 台湾海峡游泳动物种类组成及其多样性. 生物多样性, 2012, 20(1): 32-40 |
Stelzenmüller V, Maynou F, Martín P. Patterns of species and functional diversity around a coastal marine reserve: A fisheries perspective. Aquatic Conservation: Marine and Freshwater Ecosystems, 2009, 19(5): 554-565 DOI:10.1002/aqc.1003 |
Sun H, Guo ZX, Guo Y, et al. Prediction of distribution of soil Cd concentrations in Guangdong Province, China. Environmental Science, 2017, 38(5): 2111-2124 |
孙慧, 郭治兴, 郭颖, 等. 广东省土壤Cd含量空间分布预测. 环境科学, 2017, 38(5): 2111-2124 |
Sun MS, Chen ZZ, Cai YC, et al. Application of a spatial interpolation method for the assessment of fishery resources in the Beibu Gulf. Journal of Fishery Sciences of China, 2017, 24(4): 853-861 |
孙铭帅, 陈作志, 蔡研聪, 等. 空间插值法在北部湾渔业资源密度评估中的应用. 中国水产科学, 2017, 24(4): 853-861 |
Tittensor DP, Mora C, Jetz W, et al. Global patterns and predictors of marine biodiversity across taxa. Nature, 2010, 466(7310): 1098-1101 DOI:10.1038/nature09329 |
Wang JD, Lei YL, Xu KD, et al. An investigation on the biomass, abundance and distribution of meiofauna under the cold water mass and its surrounding areas of the Yellow Sea. Oceanologia et Limnologia Sinica, 2011, 42(3): 359-366 |
王家栋, 类彦立, 徐奎栋, 等. 黄海冷水团及周边海域夏初小型底栖动物现存量及空间分布研究. 海洋与湖沼, 2011, 42(3): 359-366 |
Wang P, Li H, Chen S, et al. Interpolation of permanent gully density based on slope steepness in black soil area. Research of Soil and Water Conservation, 2014, 21(6): 312-317 |
王平, 李浩, 陈帅, 等. 基于坡度的黑土区切沟密度协同克里格插值方法研究. 水土保持研究, 2014, 21(6): 312-317 |
Wang ZQ. Application of geostatistics on ecology. Beijing: Science Press, 1999
|
王政权. 地统计学及在生态学中的应用. 北京: 科学出版社, 1999
|
Whitfield AK. An estuary-association classification for the fishes of southern Africa. South African Journal of Science, 1994, 90(7): 411-417 |
Xia MH, Jia Y, Liu C. A meshless method with radial point interpolation method (RPIM). Journal of Yanshan University, 2006, 30(2): 112-117 |
夏茂辉, 贾延, 刘才. 基于径向基函数的点插值(RPIM)无网格法. 燕山大学学报, 2006, 30(2): 112-117 |
Xie Y, Chen T, Lei M, et al. Spatial distribution of soil heavy metal pollution estimated by different interpolation methods: Accuracy and uncertainty analysis. Chemosphere, 2011, 82(3): 468-476 DOI:10.1016/j.chemosphere.2010.09.053 |
Xu Y, Xian WW, Li WL. Spatial and temporal variations of invertebrate community in the Yangtze River Estuary and its adjacent waters. Biodiversity Science, 2014, 22(3): 311-319 |
徐勇, 线薇微, 李文龙. 长江口及其邻近海域春季无脊椎动物群落时空变化. 生物多样性, 2014, 22(3): 311-319 |
Yu F, Zhang ZX, Diao XY, et al. Analysis of evolution of the Huanghai Sea Cold Water Mass and its relationship with adjacent water masses. Acta Oceanologica Sinica, 2006, 28(5): 26-34 |
于非, 张志欣, 刁新源, 等. 黄海冷水团演变过程及其与邻近水团关系的分析. 海洋学报(中文版), 2006, 28(5): 26-34 |
Yu Y, Wei W, Chen LD, et al. Comparison on the methods for spatial interpolation of the annual average precipitation in the Loess Plateau region. Chinese Journal of Applied Ecology, 2015, 26(4): 999-1006 |
于洋, 卫伟, 陈利顶, 等. 黄土高原年均降水量空间插值及其方法比较. 应用生态学报, 2015, 26(4): 999-1006 |
Yue WZ, Xu JH, Xu LH. A study on spatial interpolation methods for climate variables based on geostatistics. Plateau Meteorology, 2005, 24(6): 974-980 |
岳文泽, 徐建华, 徐丽华. 基于地统计方法的气候要素空间插值研究. 高原气象, 2005, 24(6): 974-980 |
Zhang M, Cai QH, Tang T, et al. Macrobenthos community structure and its spatial distribution in Erhai watershed lakes. Chinese Journal of Ecology, 2011, 30(8): 1696-1702 |
张敏, 蔡庆华, 唐涛, 等. 洱海流域湖泊大型底栖动物群落结构及空间分布. 生态学杂志, 2011, 30(8): 1696-1702 |
Zhang Q, Zhang HT, Guo L, et al. Spatial distribution of soil heavy metal Cu content using Cokriging interpolation model combined with principal component analysis. Journal of Huazhong Agricultural University, 2016(1): 60-68 |
章清, 张海涛, 郭龙, 等. 基于主成分分析的协同克里格插值模型对土壤铜含量的空间分布预测. 华中农业大学学报, 2016(1): 60-68 |
Zhu HY, Liu SL, Jia SF. Problems of the spatial interpolation of physical geographical elements. Geographical Research, 2004, 23(4): 425-432 |
朱会义, 刘述林, 贾绍凤. 自然地理要素空间插值的几个问题. 地理研究, 2004, 23(4): 425-432 |
Zhu XH, Wu HZ, Xu FS, et al. Study on the diversity and its relational factor of the nekton community in the Yellow Sea and Bohai Sea. Acta Oceanologica Sinica, 1994, 16(3): 102-112 |
朱鑫华, 吴鹤洲, 徐凤山, 等. 黄渤海沿岸水域游泳动物群落多样性及其相关因素的研究. 海洋学报, 1994, 16(3): 102-112 |



